Lecture 5: Mapping Earth & Sky

Key Ideas:

Angular Units:

Degrees (º), Minutes ('), & Seconds of arc ('')

Terrestrial Coordinates:

Longitude & Latitude

Celestial Sphere:

Celestial Poles & Equator
Declination

Local Horizon & Zenith

Finding yourself...

Age-old questions of geography:

Where am I?
Where is someplace else?
How do I get there from here?

Ancient maps usually gave locations in terms of distances and directions from a specific place (e.g., Rome or Alexandria). This is fine for a flat earth approximation, but not obviously so good on a sphere, especially when distances get large. On spheres, it is better to use angular coordinates.

Measuring Angles

The Babylonians started the tradition of dividing the circle into 360 degrees.

360 is close to 365, the days in a year.
360 is divisible by 2, 3, 4, 5, 6, 8, 9, 10, 12, 15, 18, 20, 24, 30, 36, 40, 45, 60, 72, 90, 120 and 180 without having to use fractions.

Start by dividing the circle into quarters (90 degrees), then subdividing further using geometric constructions.

Subdividing the Degree

Degrees are divided into Minutes of Arc ('):

1 degree divided into 60 minutes of arc
"minute" from "pars minuta prima" (first small part).

Minutes are divided into Seconds of Arc ("):

1 minute divided into 60 seconds of arc
"second" from "parte minutae secundae" (second small part)
1 second = 1/3600th of a degree (very small)

Question: Why 60?

Answer: The Babylonians (again)...

60 is divisible by 2, 3, 4, 5, 6, 10, 12, 15, 20, and 30 without fractions.

The Babylonians actually subdivided the degree as fractions of 60, for example:

7 ¹⁴_/60 degrees

Claudius Ptolemy introduced the modern notation of expressing angles in terms of minutes and seconds of arc

7º 14' 00"

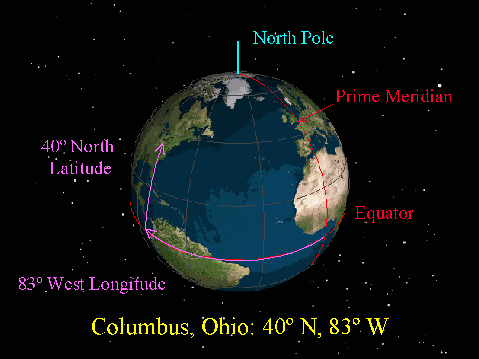
Angular Coordinates on Spheres

Since the Earth's surface is approximately spherical, we divide the surface into a grid of arcs rather than a rectangular grid.

(Click on the image to view at full scale [Size: 51Kb])

Equator:

Great circle dividing a sphere into to Equal North and South halves.
The Equator runs East-to-West

Meridian:

Great Circle passing through the North and South Poles, through a location on the sphere.
Crosses the Equator at Right Angles, and runs North-to-South.

Prime Meridian:

Special Meridian that defines Zero Longitude.
Runs through Greenwich England by current international agreement (1884).

Longitude:

Angle East or West along the Equator from Prime Meridian.

Latitude:

Angle North or South along the Meridian from the Equator.

Lost & Found

The system of Latitude and Longitude was invented (or at least brought into its classic form) by Claudius Ptolemy (c 140AD), the "Father of Modern Geography".

It was all but forgotten in Europe after the collapse of the Roman Empire:

Flat Earth maps dominate through the middle ages.
The most famous are the "T-O" maps centered on Jerusalem.

Ptolemy was rediscovered, with the Spherical Earth, about 1300:

Prime Meridian is now in Greenwich England instead of the "Fortunate Isles" of Ptolemaic tradition (probably the Canary Islands).

The modern system of latitude and longitude is largely the same as Ptolemy's except for the details.

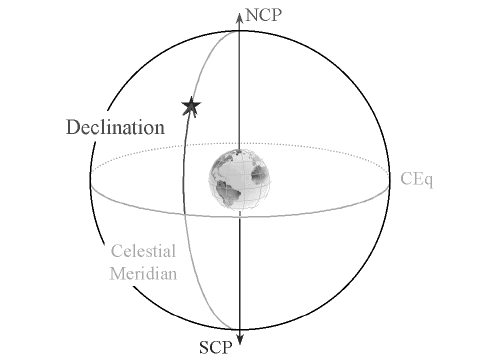
The Celestial Sphere

The Sun, Moon, and Stars are so far away, we cannot perceive their relative distances as depth in the sky. Instead, they appear to be projected onto a Celestial Sphere centered on the Earth.

(Click on the image to view at full scale [Size: 20Kb])

Celestial Equator:

Projection of the Earth's Equator onto the sky.

Celestial North & South Poles:

Intersection of Earth's Poles with the sky

Celestial Meridian:

Great Circle passing North-South through the North Celestial Pole (NCP) and South Celestial Pole (SCP) on the sky.

Declination:

The celestial equivalent of Latitude is called Declination.
Declination is the angle North or South along a celestial meridian from the celestial equator to the object (e.g., star).
Measured in degrees from the Celestial Equator

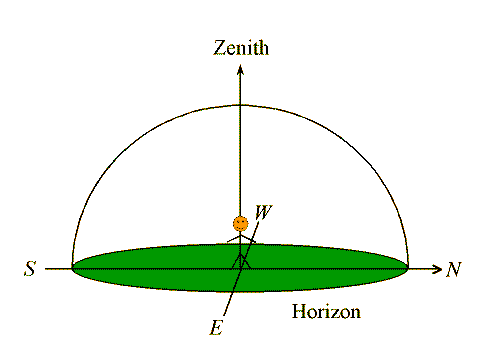
The Local Sky

From any particular location on the surface of the Earth, we can only see half of the sky at any instant:

One half stretches overhead to the Horizon.
Other half of the sky is below the Horizon.

In addition to the Horizon, we define a few specific points on the sky:

Zenith:: The point directly overhead.
Nadir:: The point opposite the Zenith, directly below your feet.
Cardinal ("Compass") Points:: The 4 cardinal directions: North, South, East and West.

(Click on the image to view at full scale [Size: 8Kb])

What part of the Celestial Sphere you can see depends on

Where you are on the Earth (Latitude & Long)
What time it is (date and time)

The overall effect is that we see objects rise above the Eastern Horizon, and set below the Western Horizon as the Earth Rotates.

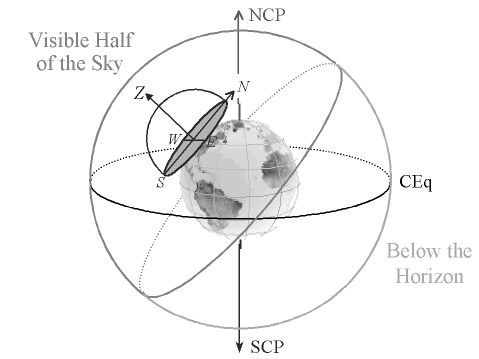
(Click on the image to view at full scale [Size: 33Kb])

Here we see the local sky "dome" placed on the Earth at the position of Columbus, Ohio, with the Celestial Sphere drawn. The visible half of the sky at this instant is shown in green, while the part of the sky below the horizon (and so invisible) at this instant is shown in red. As the Earth rotates towards the east, those parts of the sky just below the eastern horizon will rise in the east, while those just above the western horizon will set.

Celestial Navigation (part way)

The Angle Between the North Celestial Pole and the North Compass Point on the Horizon is your Latitude!

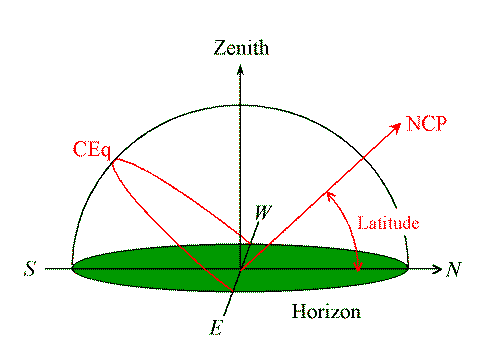
(Click on the image to view at full scale [Size: 11Kb])

In principle, if you can measure the altitude of Polaris, you are measuring your Latitude to a precision of about 1 degree.

Variants on this technique are at the heart of the practice of Celestial Navigation for deterimining Latitude today.

Longitude depends on the time, and is much harder to measure (and is another story for another day).

Return to [ Unit 2 Index | Astronomy 161 Main Page ]