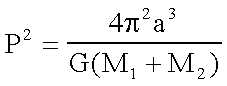Astronomy 1144: Introduction to Stars, Galaxies, and Cosmology
Todd Thompson
Department of Astronomy
The Ohio State University
Lecture 7: Stars III: Binaries
- Measure stellar masses from binary stars
- Only way to measure stellar masses
- Only measured for ~150 stars
- Types of Binary Stars:
- Visual
- Spectroscopic
- Eclipsing
- Stellar radii have been measured for ~600 stars
FGrav=GM1M2/d2
P2=4 &pi2a3/[G(M1+M2)]
Vcirc=(GM/r)1/2
Measuring Masses
Masses are measured by using the effects of gravity on objects:
Examples:
- Your mass from how much the Earth's gravity pulls upon you
(your "weight").
- Earth's mass from the orbital motionss of the Moon or
man-made satellites.
- Sun's mass from the orbital motions of the planets.
Binary Stars
Apparent Binary Stars:
- Chance projection of two distinct stars along the line of sight.
- Often at very different distances.
True Binary Stars:
- A pair of stars bound together by gravity.
- Orbit each other about their common center of mass.
- About 60% all systems have 2 or more stars
Types of True Binaries
Visual Binary:
- Can see both stars and follow their orbits over time.
Spectroscopic Binary:
- Too close to see as separate stars, but you can detect
their orbit motions by the Doppler shifts of their spectral lines.
Eclipsing Binary:
- Too close see as separate stars, but we see the total brightness
of the system decrease when the stars periodically eclipse each other.
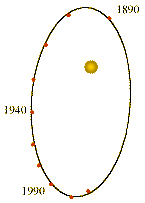
Visual Binaries
Two stars orbiting about their center-of-mass.
(Graphic by R. Pogge)
Center of Mass
Two stars orbit about their common center of mass.

(Graphic by R. Pogge)
- Measure semi-major axis, a, from projected orbit & the
distance.
- Relative positions about the center give:
M1/M2 = a2/a1
Here are two movies showing simulations
of visual binary stars, one with a circular orbit and the other with
an elliptical orbit. These movies emphasize the point about their
orbiting around their common center of mass in all cases.
See this link for a picture of Sirius and its companion Sirius B.
Measuring Masses
Recall Newton's Version of
Kepler's Third Law:
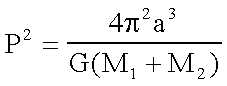
(Graphic by R. Pogge)
Procedure:
- Measure the period, P, by following the orbit.
- Measure semi-major axis, a, from the observed angular separation
between the two stars and the distance to the system.
- Solve the formula above to get the Total Mass (M1+M2)
- Estimate the Mass Ratio, M1/M2, from the projected orbit on the sky and the center-of-mass
- Solve for the individual masses.
Problems:
We need to follow an orbit long enough to trace it out in detail:
- This can take decades
- Need to work out the projection on the sky
Measurements depend on knowing the distance:
- semi-major axis depends on d
- derived mass depends on d3
Small errors add up quickly (10% error in distance translates into
a 30% error in the mass!).
Spectroscopic Binaries
Most binaries are too far away to be able to see both stars separately.
But, you can detect their orbital motions by the periodic
Doppler shifts of the spectral lines:
- Determine the orbit period & size from the pattern of
orbital velocities

(Graphic by R. Pogge)
Here is a movie showing a simulation of
a spectroscopic binary star.
Problems:
Often cannot see the two stars separately:
- Semi-major axis must be estimated from the orbit parameters.
- Can't tell how the orbit is tilted on the sky: are we viewing
the binary edge-on, or face-on, or in between?
Everything depends critically on knowing the distance.
Eclipsing Binaries
Two stars orbiting nearly edge-on to our line-of-sight. This
is a special case and solves the problem mentioned
above for spectroscopic binaries.
- See a periodic drop in the total brightness of the system
as one star eclipses the other.
- Combine with spectra (Doppler shift) to measure orbital speeds with time.
With the best data, one can find the masses of the stars
without having to know the distance!!!
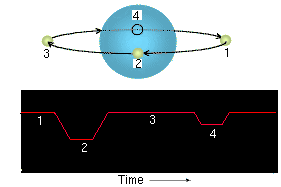
(Graphic by R. Pogge)
Here is a movie showing a simulation
of an eclipsing binary star and its light curve.
Problems
Eclipsing Binary stars are very rare.
Measurement of the light curves is complicated by details:
- Partial eclipses yield less accurate numbers.
- The atmospheres of the stars soften the edges.
- Close binaries can be tidally distorted.
Despite this, most of the best stellar masses are from eclipsing binary
systems.
Additionally, from the shape and duration of
the eclipses we can also determine the radii of the
stars.
Stellar Masses
From a combination of visual and eclipsing binaries, masses are
known for about 150 stars.
- Range: ~0.07 to 60 Solar Masses
A few very massive stars are known, with masses of 80-120 Solar Masses,
but these are very unusual and rare.
We will explore the masses of stars more in the next unit on stellar
structure.
Stellar Radii
Radii are very difficult to measure because stars are so far away.
For example, from a distance of 1pc, the Sun is only 0.0093 arcseconds
in diameter.
Methods:
- Interferometry (single stars)
- Lunar Occultation (single stars)
- Eclipsing binaries (need distance)
The details of these techniques are beyond the scope of this course to
discuss.
Stellar Radii have been measured for about 600 stars so far. The large
number compared to a few years ago is due to advances in techniques like
optical interferometry.
Follow this link to see a picture of Betelgeuse, the bright red star that is the right shoulder of the Orion constellation.
Updated 8/25/2013 by Todd Thompson
Original Version by R. Pogge.